A Student's Guide to Cost Benefit Analysis for Natural Resources
Lesson 4 - The Mechanics of
Discounting
Interest and Discount Rates
The purpose of cost-benefit analysis (CBA) is to determine economic merit of public investment projects. Because these projects almost always occur over several years, they will have cost and benefit flows which may occur very far apart in time. For this reason, we cannot simply add or subtract cost and benefits which occur at different points in time. We must use discounting.
Now, we will discuss the arithmetic of compounding and discounting:
Compounding: Computing the earnings on money carried forward in time. You invest an initial amount, (i.e., the principal.) This principal earns interest. As the process moves through time, you earn interest on both the principal and the accumulated interest.
Compound interest formula:
![]()
where; Vn is the value of a sum in some future year (n), Vo is the value of a sum in the present year, t = no. of intervening periods between n and o; i = interest rate. We normally say for convenience that dollar outflows or inflows occur at the end of a period.
Compounding example: Given an interest rate, the number of time periods and a present value we can compute a future value w/compound interest:
(1) Vo= $12,000, i = .05, t = 8 years. Calculate Vn.
Vn = $17,729.47.
You can compound yearly, quarterly, monthly, daily. The more frequently you compound the more interest you earn, but often the increase is not much. Banks often use this as a sales gimmick. For example, quarterly compounding, you simply multiply the number of years by 4 and divide the interest rate by 4; then use the compounding formula. Example:
Vo=$12,000, i=.05/4=.0125, t=4x8=32 quarters. Calculate Vn.
Vn = $17,857.57
The most extreme compounding case is continuous compounding. You see this frequently in the science literature:
Vn=Voert
e= 2.71828; base of natural logs.
Vo= $12,000, i = .05, t = 8 years. Calculate Vn.
$17,901.89.
Discounting is the reverse of compounding. We reduce a future value to a present value by discounting. Discounting is an important concept for CBA; it makes cashflows occurring at different times algebraically comparable.
Present (i.e., discounted) value is sometimes referred to as the capitalized value. We now call the interest rate the discount rate, but we will still use the same symbol "i". For government (social) projects, we will later call this discount rate the Social Discount Rate.
Discounting formula:
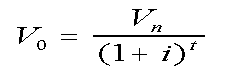
Example of discounting:
Vn= $17,730, i=.05, t=8 years. Calculate Vo.
Vo= $12,000.36. The same as before except for rounding.
Discounting with a positive discount rate always will reduce the size of the initial value.
The Earnings Formula:
This is a formula which can be derived from the compound interest formula and which can tell you the annual percentage rate of earning on an investment. You simply solve the compound interest formula for "i". It computes the mean of a geometric series (i.e., a geometric mean) as opposed to an arithmetic mean. The earnings formula:
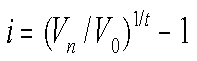
In other words, if you knew how much was initially invested, and how much value how accumulated after n years, you could solve for "i" -- the annual rate of earning. Often, this is of interest to an investor: what has been my annual rate of earning, or of asset appreciation, over time?
Example: You bought a house for $85,000 and sold it 12 years later for $125,000. What was the annual rate of value appreciation for the house?
V0=$85,000, Vn=$125,000, t=12 years. Solve for i?
i=.03266 = 3.2%/year. This is the geometric mean.
If you had computed a simple arithmetic mean you would have obtained:
$125,000 - $85,000 = $40,000
$40,000/$85,000 x 100 = 47%
47%/12 years = about 3.9 %/year
Is this wrong? Let's just say the geometric mean is more meaningful. The arithmetic mean will always be larger than geometric mean.
Impact of Time and Discount Rates on Discounted Values
Time can have a major impact on discounted future values. Let's illustrate this by taking our previous Vn ($17,730) and
i = .05. Now discounting for a period of 25 years and look at the present value result.
Vo = $5235.72
Now let's discount for a period of 100 years and look at the result.
Vn= $17,730, i = .05, t=100
Vo=$134.83
The value is substantially diminished by time.
High discount rates will have a similar impact on discounted values; distant values are substantially diminished. What are the implications for long-term public projects where benefits are received in the distant future? Benefits can get "clobbered" by time and discount rates. It is the bane of those wanting to justify a long-term project. We conclude that time and discount rates can have a major impact on present value calculations
Nominal and Real Discount Rates and Inflation
In economic analyses, you will encounter the terms real interest rates and nominal interest rates. Nominal rates are what you observe in the actual world, (this is confusing!) i.e., the market rates (hence nominal rate is sometimes called market rate.) For example, the annual percent earning rate of stocks, Treasury Bills, Certificates of Deposit, Bonds. These observable market rates are called nominal interest rates.
Nominal interest/discount rates are composed of:
1. real rate of return
2. measure of inflation
Real interest rates, in contrast to nominal rates, do not include inflation. Real rates are called real because they represent real economic sector changes in demand/supply. By real economic sector, we mean the household and business sectors of the economy in contrast to the monetary sector.
Inflation is defined as a rise in the general level of prices. The monetary sector of the economy said to be the fundamental cause of inflation; too much money chasing too few goods. Inflation usually happens when the money supply expands rapidly. With money to spend, economic demand is strong so that shortages of labor and materials become widespread. Thus prices in general rise, and money starts losing its purchasing power.
Real interest rates are not directly observable. They must be calculated by removing the effects of inflation from the nominal rates. An approximation of the real interest rate is provided by:
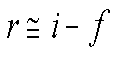
say if: i = 10% (.01), f = 4% (.04)
then r ..10 - .04 = .06
where: r = % real interest rate for an investment of a certain level of risk; i = % nominal rate; f = % rate of inflation.
Many people use this simple subtraction method to get real discount rates:
Quotes:
1. "From annual nominal rates of return, annual percentage changes in the CPI were deducted and real rates were averaged." Holland and Myers
2. US Forest Service subtracted changes in the GNP implicit price deflator from nominal (AAA bond) rates. Then arithmetically average several years of these real rates to get 4%.
3. "I use the 12‑month change in the Consumer Price Index as the rate of inflation. If a note has a nominal yield of 5% while the inflation rate is 3%, the real yield is 5 minus 3, equaling 2%." Professional investor.
However, the true (precise) relationship of real (r), nominal (i), and inflation (f) rates is:
1+i = (1+r)(1+f)
multiplying RHS terms yields:
1+i = 1+r+f+rf
solving algebraically for r (the real rate, what we want to know):
r= [(1+i)/(1+f)]-1
Compare the approximation formula with the precise formula, given that i=.10, f=.04:
![]()
.06=.10-04.
-and-
r= [(1+i)/(1+f)]-1
.0577 = [(1.1)/(1.04)]-1
There is a slight difference.
Where do you obtain nominal interest rates and rates of inflation?
1. Nominal rates are directly observed in the market place. One source is the historical averages of rates of return on investment (ROI) for various investment vehicles of varying risk.
Investment Returns 1926‑1996, Average Annual Return:
1. Stocks (S&P 500 Index) +10.7%
2. Bonds (Long‑term U.S. Government) +5.1%
3. U.S. Treasury bills +3.7%
These are nominal returns. Return for various risk levels is reflected in the nominal rates.